We are always interacting with ratios often without realizing it. From comparing the heights of different people to comparing crowd sizes, ratios are an everyday experience.
Ratios are a useful way of comparing two or more quantities of different things in order to better understand how they relate to each other. They can be expressed mathematically to give a precise picture of proportions.
Finding ratio is simply calculated by dividing one number with another, often expressed as a fraction. Each number in the fraction corresponds to the quantity of the items you are comparing.
The top number of the fraction and its bottom number can be written together with a colon symbol (:) separating them.
For example, 3 cows and 4 goats give a fraction of ¾, which can be written as 3:4.
The ratio of the circumference of a circle to its diameter gives us the very familiar number we know of 22/7, 22:7, or 3.14.
Let’s look at 10 examples of ratios that are commonly seen in daily life.
1. Food Recipes
Preparing food involves a very careful process. Each food or dish type needs a particular mix of ingredients.
Add too much of any of these or too little and the food will taste awful. So, it is crucial to get the mix just right. Here are two examples of food recipes.
For the base used for vinaigrette, you need a solution that is 1 part vinegar to 3 parts of oil. This can be expressed as 3:1.
Brine is a salty solution that can be used as a base for cooking poultry or pork. The recipe used is 20 parts of water for every part of salt. This can be written as 20:1.
Note that by parts, we mean using the same unit consistently for each ingredient. For example, you can use 1 ounce of vinegar and 3 ounces of oil to make the vinaigrette.
2. Gender Mix of a Class
Most classes have both boys and girls. You can calculate how their numbers compare with each other using ratios.
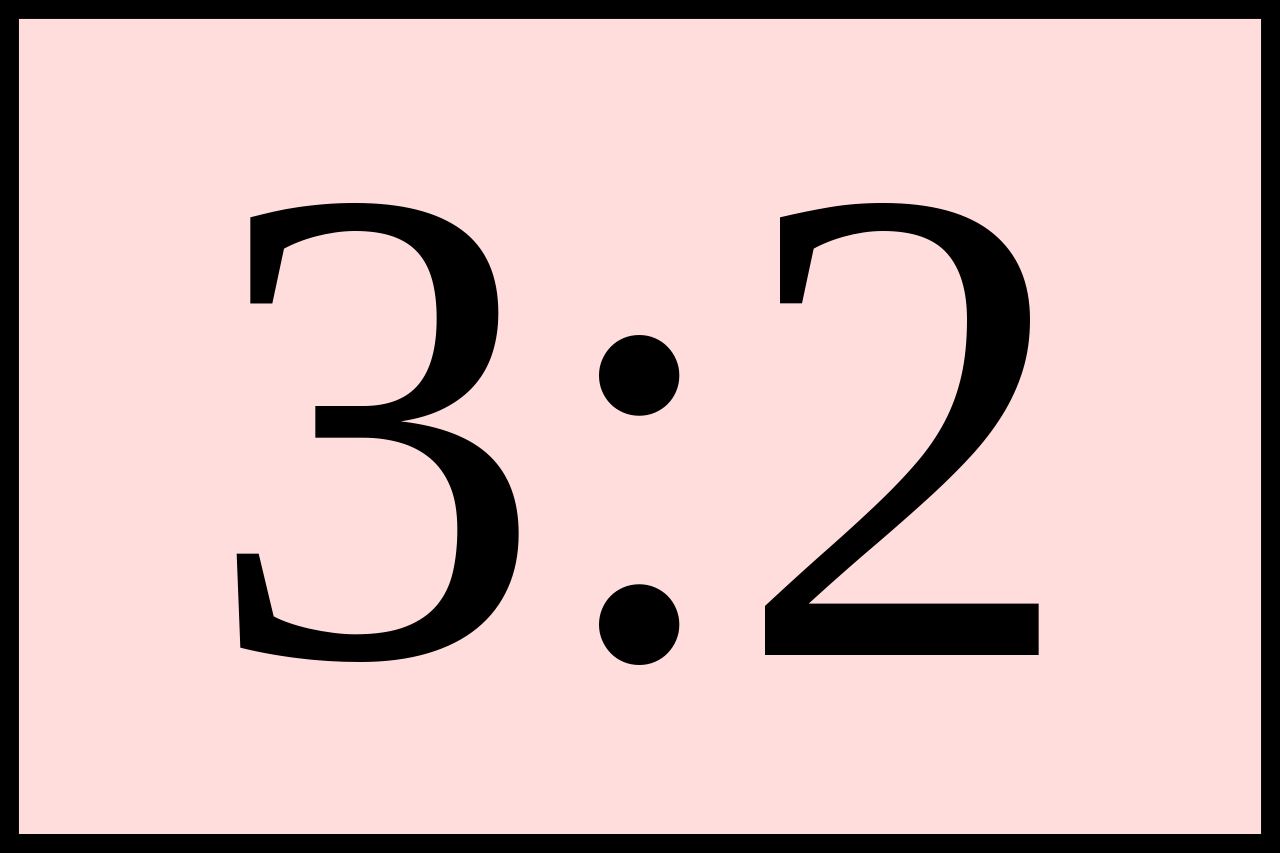
For example, if they are 60 girls and 40 boys in the class, the ratio of girls to boys can be found by dividing 60 by 40.
60/40 = 3/2. This can be expressed as 3:2. (Always simplify to the lowest terms). This means that for every 2 boys, there are 3 girls in this class.
3. Lottery Win Chances
Lottery games are games that involve probabilities. This can be expressed as a fraction or ratio.
For example, if you play a lottery game that gives you a 1 in 2,000,000 chance of winning, this can be expressed as 1/2,000,000 or 1:2,000,000.
This gives you a very small chance of winning each time you play of just 1 out of 2,000,000 attempts.
4. Comparing Speeds
How long will it take you to journey between two cities? This depends on what you are using for your travel.
For example, you will be traveling at about 100 mph if you are using a car but only 30 mph if you are using a horse.
This means the speed ratio of the horse to the car is 30/100 or 3:10.
The car is much faster than the horse, so you will arrive earlier to your destination.
5. Comparing Prices
When you are buying your groceries, you probably need to compare the prices at different stores so that you can decide where to buy at a cheaper price.
For example, if a loaf of bread costs $3 at store A and $4 at store B, the price ratio of store A to B is ¾ or 3:4.
Buying bread at Store B is more expensive.
6. Comparing Weights
We don’t all weigh all the same. Even among our peers or age mates, weights can vary considerably.
It can be interesting to mathematically compare these weights which can be conveniently done through ratios.
Here is an example:
Peter weighs 65 kg on the scales. His friend, David, weighs 105 kg. You can calculate ratios by dividing 65 and 105.
65/105 =13/21 (Again make sure to reduce the numbers to their lowest terms by dividing through with 5)
This gives a ratio of 13:21. So, Peter’s weight ratio to David is 13:21.
7. 22/7 or 3.14 used in circles
We have already indicated that the ratio of a circumference to the diameter of any circle can be compared using a ratio, which gives the well-known result of 22/7 or 3.14.
Let’s look at an actual example of this.
The diameter of a circle is 14 cm. Therefore, its circumference is:
22/7 x 14 = 44 cm
The circumference (44cm) can now be compared with the diameter in this way:
44/14 =22/7 which can be written in ratio form as 22:7
This confirms to us that the ratio of the circumference to the diameter is 22:7
8. Mixing Cement for Building
When constructing buildings, we often need to mix cement and sand to produce a mortar mix.
This material acts like glue to bind parts of your building together. The mixing should be done with the right quantities so that the mortar is effective to make your building strong.
The recommended mixing ratio is 3 parts of sand to 1 part of cement or 3:1.
This means that for every 3 equally sized scoops you take of your sand, you should take 1 scoop of cement.
9. Comparing Currencies
Different countries use different currencies for buying things.
For example, the US uses the US dollar while a country like Kenya uses Kenyan Shillings.
The values of the different currencies are rarely the same. For example, $1 may be equal to 100 Kenyan Shillings. This can be written in ratios to compare them.
$1=Ksh 100. Therefore, the ratio is 1/100 or 1:100.
10. Conversion between Metric and US measuring units
So far, we have used examples where we only use whole numbers to represent ratios. In our final example, we show a situation often met in life where we can also use decimal numbers.
Metric and US standards are two different ways that can be used for measuring different things. Using ratios makes it easy to convert your measurements from one system to another.
1 pound approximately equals 0.454 kilograms. This gives a ratio of 1:0.454.
So for every pound you weigh, this approximately equals 0.454 kilograms.
To convert your pounds to kilograms, we only have to multiply by 0.454.
For example, if you weigh 300 pounds then in kilograms you weigh 136.2 kg (300 x 0.454)
Conclusion
There you have it; Detailed examples of ratios in daily life.
The use of ratios has simplified how we view comparisons of different quantities.
They give you a better interpretation of proportions and quantities, leading to better decision-making in life.
